
 |
Intermediate Macroeconomics | |
3. Long-Run Economic Growth |
An improving standard of living depends on economic growth. To consume more we have to produce more. We will investigate three key questions in this chapter:
1. What determines the growth rate of output over time?
2. Why do poor countries remain poor and can they ever catch up?
3. Is there an optimal rate of economic growth?
We will attempt to address these questions through two complementary lines of research. First we develop a growth accounting approach that will let us identify what has contributed to historical growth. Second we will present two different macroeconomic growth models that try to explain differences in growth and income across countries and provide insight into the desired path of growth.
Economic growth and our standard of living typically are measured by the quantity of goods and services we consume. The best available economic measure of quantity is real GDP. Real GDP eliminates the effects of inflation. A country's nominal GDP may be growing at 20 percent a year, but if its inflation rate is 30 percent a year then its actual output or real GDP is in fact shrinking. Real GDP may not be a perfect indicator of our well-being because it ignores some of the unmeasured benefits and costs of our behavior that we discussed in the earlier chapter on GDP accounting. But it is the best indicator that has been consistently measured over time.
Economic growth is generally measured in one of two ways. First, when we look at two or more countries we can compare total or aggregate real GDP. This measure doesn't accomplish much more than showing that one economy is larger or smaller than another or that it is growing faster or slower. Aggregate real GDP is of limited use because it does not reveal whether the residents of a given country are better or worse off. One country's aggregate real GDP may be twice that of another, but if it has four times the population (or labor force) then each person produces only half as much. But size does matter when you are considering possible economies of scale (larger is often better) or resources (capital and labor) that are available to the economy.
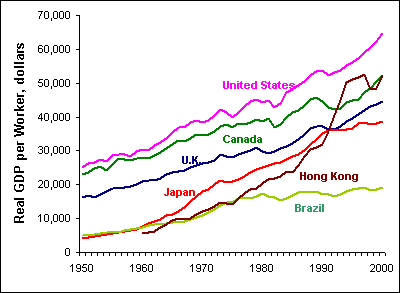
The second measure of economic growth is real GDP per person or per worker or per labor hour. A per capita real GDP provides a measure of the average output of each person. If each person produces more (on average) then each person can presumably consume more and is better off. For example, Figure 3-1 presents trends in the economic growth of real GDP per worker of six industrialized countries over the last 50 years. Growth is measured as real GDP per worker to eliminate the effects of inflation and population growth. U.S. aggregate nominal GDP grew an average 7.3% per year between 1950 and 2000. Real GDP, which eliminates price inflation grew by an average 3.4% per year over this period. Part of the growth in real output is due to a growing population and labor force. Dividing real GDP by the employed labor force produces an average growth rate in real GDP per worker in the U.S. of 1.9% per year. Thus we can directly compare the well-being of the residents of each country and the growth in the productive capacity of the average worker of both very large (the U.S.) and small (Hong Kong) economies.
| Economic Growth - change in aggregate real GDP or average real GDP per person over time.
Standard of Living - the real value of the quantity of goods and services consumed by the average person, typically measured as average real GDP per person, per worker, or per family. |
Japan started the second half of the 20th century with a real GDP per worker at less than one-fourth that of the United Kingdom. But an impressive real annual growth rate per worker of almost 5.6% per year allowed Japan to catch the U.K. by 1990. Japan's engine of growth ran out of steam in the last 10 years of the 20th century with an average growth rate of 1.0%. Hong Kong did even better than Japan, almost catching the United States by 1997 before stalling.
| Table 3-1. Economic Growth of Six Countries by Decade | ||||||
| United Kingdom |
United States |
Japan | Canada | Brazil | Hong Kong | |
| Average 1950 - 1960 | 2.55% | 1.94% | 6.64% | 1.96% | 4.08% | n.a. |
| Average 1960 - 1970 | 2.30% | 2.40% | 8.94% | 2.21% | 4.08% | 7.79% |
| Average 1970 - 1980 | 1.31% | 1.41% | 3.39% | 1.07% | 4.73% | 5.24% |
| Average 1980 - 1990 | 2.17% | 2.00% | 3.34% | 1.49% | -0.29% | 4.69% |
| Average 1990 - 2000 | 1.87% | 1.82% | 1.00% | 1.57% | 1.24% | 5.00% |
| Average 1950 - 2000 | 2.04% | 1.91% | 4.62% | 1.66% | 2.75% | n.a. |
| Note: Average growth rates are compounded annual average growth rates in real GDP per worker. Source: Penn World Tables (http://datacentre.chass.utoronto.ca/pwt/alphacountries.html). | ||||||
While increases in the average standard of living from year to year may appear small, differences between generations can be great. Small changes build over time. For example, from 1950 to 2000 the difference between the growth rates of real GDP per worker for the United States and Canada was only 0.25% (Table 3-1). This small difference in growth rates led to a widening of the lead the U.S. had in real GDP per worker from about $2,000 per worker in 1950 to over $12,000 in 2000 (Figure 3-1).
![]()
Understanding why economies grow begins with the production function. The production function describes the relationship between the inputs of labor and capital and the output of goods and services. Growth in aggregate output is then explained by the following four factors:
The problem we face is how do we attribute the historical growth in aggregate output to changes in the quantities and qualities of capital and labor. Measuring the quantity of capital and the quantity of labor is not a serious problem. There is published data on the dollar value of installed capital and the number of labor hours worked. Measuring qualities, however, is a problem. Rather than try to identify and separate the quality of capital from the quality of labor, economists calculate a combined productivity index. The productivity index is referred to as multifactor productivity (also called total factor productivity), which represents output from the "factors" of production, capital and labor. Growth in multifactor productivity represents an increase in output that results from improvements in production processes, whether due to improvements in the quality of capital (such as from new technology) or improvements in the quality of labor (such as from better education or training), with the quantities of all inputs unchanged.
| Multifactor Productivity - productivity is a measure of economic efficiency which shows how effectively economic inputs are converted into output. Multifactor productivity is measured by comparing the amount of goods and services produced with the factors (e.g., capital and labor) used in production. |
We can explain historical growth in output in terms of changes in the quantity of labor, the quantity of capital, and multifactor productivity by starting with a general representation of the production function shown in equation (1). Production is a function of the economy's use of capital, K, labor, L, and a multifactor productivity index, A.
| Y = A • f(K, L ) | (1) |
We can convert equation (1) into a growth accounting equation (2), which relates the growth rate in aggregate output to the growth rates of capital, labor, and multifactor productivity (see Appendix A for details of the derivation).
| ΔY = ΔA + εK ΔK + εL ΔL Y A K L |
(2) |
A growth rate is simply the amount of increase or decrease divided by the starting level. For example, let's say last year we produced 100 widgets and this year we produced 101 widgets. The increase in widget production (ΔY = 1) divided by last year's total production (Y = 100) equals a growth rate (ΔY / Y) of 0.01, or 1 percent (0.01 x 100).
The elasticity of output with respect to capital, εK, is the percent change in output that results from a 1% change in the level of capital with all other variables remaining unchanged. The elasticity of output with respect to labor, εL, is the percent change in output that results from a 1% change in the total labor force employed with all other variables remaining unchanged. For example, if the elasticity of output with respect to labor is 0.7, a 1% increase in the number of workers or labor hours will increase aggregate output by 0.7%.
If we know the elasticities of output with respect to capital and to labor we can empirically measure the relative importance of each of these sources of growth. Consider a simple example. Assume multifactor productivity, the level of real capital, and the labor force are all growing at 1% per year and the elasticity of output with respect to capital is 0.3 and the elasticity of output with respect to labor is 0.7. We can calculate the growth of aggregate output as ΔY/Y = 1% + (0.3 • 1%) + (0.7 • 1%) = 2%.
Now consider what happens if one of the three growth rates is 2% rather than 1%. Growth in multifactor productivity of 2% would boost aggregate output growth from 2% to 3%. Additional growth in the level of capital of 1% would add 0.3% to the output growth rate, and an additional 1% growth in the labor force would add 0.7% to output growth.
![]()
An empirical study is a test of a hypothesis or theory using actual data. For example, we may want to empirically test the hypothesis that the U.S. economy grows faster during the year before a Presidential election than other countries and grows slower in the year following. The empirical test would involve entering economic growth rates for selected countries in a spreadsheet and statistically comparing growth rates for those two groups of years.
Comparing growth rates across countries as in Table 3-1 is not a problem despite differences in currencies because growth rates are independent of the units of measurement. But when we compare levels of output or income across countries as in Figure 3-1 we run into the problem of differences in currencies. U.S. output is measured in dollars and Japanese output is measured in yen. The first step in comparing levels is to convert currencies using foreign exchange rates. Output in yen can then be expressed as output in dollars by multiplying by the dollar-yen exchange rate.
We still have one significant problem - differences in the cost of living. For example, if we express welfare in terms of income per worker the income in dollars per worker in Japan may be identical to that of the United States. However, if the cost of living in Japan is higher because of housing, food and other costs, this direct comparison of incomes does not reveal actual differences in purchasing power and the standard of living.
Let's compare two U.S. States, which avoids the small complication of converting different currencies using exchange rates. The median annual household income in Virginia in 2000 was $46,677, while that of Mississippi was $31,330. Does this mean the average household in Virginia was better off? Not necessarily because the median value of owner-occupied housing in 2000 was $125,000 in Virginia versus $71,400 in Mississippi. Incomes may be lower on average in Mississippi but the cost of living, at least in terms of housing, was also lower. Output and income levels must be corrected for purchasing power parity in order to make valid comparisons.
| Exchange Rate - The price of one currency in terms of another currency. For example, the exchange rate between the yen and the dollar may be 100 yen = $1.00. This means that you need to pay a price of 100 yen to get $1.00, or pay $1.00 in exchange for 100 yen. Exchange rates can be fixed or floating. Fixed means that they stay at the same value as set by the government. Floating means that they fluctuate day to day according to the market.
Purchasing Power Parity (PPP) - The PPP exchange rate is represents the quantities of money in each currency that would buy exactly the same basket of goods in both countries. For example, say a certain basket of goods costs 1,000 Yen in Japan, and the same basket costs $10 in the U.S.. The PPP rate would be 100 Yen = $1. The PPP exchange rate will often differ from the actual exchange rate. |
International comparisons are made easier using data provided by Alan Heston, Robert Summers and Bettina Aten with the Center for International Comparisons at the University of Pennsylvania. The Penn World Tables (http://pwt.econ.upenn.edu/) provide real national income accounts converted to U.S dollars based on purchasing power parity for 179 countries for some or all of the years 1950-2000. The Organization for Economic Cooperation and Development (OECD) also lists PPP exchange rates for its member countries (Annual National Accounts for OECD Member Countries, http://www.oecd.org/).
The Japan-U.S. exchange rate makes an interesting study of the role of purchasing power parity. In 1980 the market exchange rate was 227 yen to the dollar. The PPP exchange rate (based on a basket of goods and services represented by GDP) was close to the actual at 231 yen to the dollar. The exchange rates were very different in 2000: the actual rate was 108 yen per dollar and the PPP exchange rate was 155 yen per dollar. While the yen had appreciated in value over those 20 years (it now takes fewer yen to buy one dollar), the smaller decline in the PPP exchange rate indicates that it has become relatively more costly to live in Japan, which has offset some of their gain in the actual exchange rate.
The growth accounting equation (2) gives us a foundation for evaluating historical economic growth and its causes. Empirical studies of economic growth generally follow three steps:
1. Determine the growth rates of aggregate output (ΔY/Y), labor (ΔL/L), and capital (ΔK/K) over some period of time.
2. Estimate the elasticities of output with respect to capital, εK, and to labor, εL. This is made somewhat easier if we assume markets are competitive, which implies that the elasticities are equal to income shares that are observable (see Appendix B). For the United States the elasticity of output with respect to capital has been about 0.3, and the elasticity of output with respect to labor about 0.7.
3. Calculate the contribution of the growth in capital (εK ΔK/K) and the growth in labor (εL ΔL/L) to the growth in output (ΔY/Y). The difference between the growth in aggregate output and the contributions from capital and labor is attributed to multifactor productivity change. Productivity change is treated as an unexplained residual.
Two well known studies that follow this growth accounting procedure are those of Robert Solow and Edward Denison, which are summarized in Table 3-2. One interesting result of these studies is the smaller contribution made by the growth in capital. Increasing aggregate output depends more on increases in multifactor productivity than on real capital. Denison suggested that almost two-thirds of the increase in multifactor productivity (0.66% of the 1.02%) was due to advances in knowledge. Improved resource allocation (e.g., movement of workers from farms to the cities) and economies of scale added 0.23% and 0.26% to output growth, respectively.
| Table 3-2. Solow and Denison Studies of U.S. Growth | ||
| percent change per year | ||
| Solow | Denison | |
| Period Covered | 1909 - 1949 | 1929 - 1982 |
| Total Output, ΔY/Y | 2.9 | 2.92 |
| Capital Inputs, ΔK/K | 0.32 | 0.56 |
| Labor Inputs, ΔL/L | 1.09 | 1.34 |
| multifactor Productivity, ΔA/A | 1.49 | 1.02 |
| Sources: Robert Solow, "Technical Change and the Aggregate Production Function", Review of Economics and Statistics, August 1957; Edward F. Denison, Trends in American Economic Growth, The Brookings Institution, 1985. | ||
We can take our own stab at estimating the growth in multifactor productivity using published data on real GDP, hours worked and the private capital stock. For example, Table 3-3 presents these data published by the Bureau of Economic Analysis (Dept. of Commerce) and Bureau of Labor Statistics (Dept. of Labor).
| Table 3-3. U.S. Growth Accounting | |||
| 1987 | 2003 | Annual Average Percent Change | |
| Output: Real GDP Quantity Index (2000=100) | 65.958 | 105.749 | 2.99 % |
| Capital: Private Nonresidential Fixed Assets Index (2000=100) | 69.663 | 105.714 | 2.64 % |
| Labor: Total Private Aggregate Weekly Hours Index (2002=100) | 79.77 | 98.62 | 1.33 % |
| Sources: Real GDP from Bureau of Economic Analysis (BEA), National Income and Product Accounts, Table 1.1.3 (http://www.bea.gov/bea/dn/nipaweb/index.asp); Nonresidential fixed assets from BEA, Fixed Assets, Table 4.2 (http://www.bea.gov/bea/dn/FA2004/SelectTable.asp); and Total private aggregate weekly hours from Bureau of Labor Statistics, Current Employment Statistics Survey, Series CES0500000040 (http://www.bls.gov/ces/home.htm) | |||
Given the estimates for the elasticities of output with respect to capital (εK=0.3) and labor (εL=0.7) published by others (or we could calculate income shares as explained in the Appendix) we can easily calculate the growth in multifactor productivity:
| Real GDP growth rate = Productivity growth rate + εK • fixed assets growth rate + εL • labor growth rate | |
| 2.99 = Productivity growth rate + 0.3 • 2.64 + 0.7 • 1.33 | |
| Productivity growth rate = 2.99 - 0.79 - 0.93 | |
| Productivity growth rate = 1.27 % per year average |
We calculate an average growth rate of multifactor productivity of 1.27 percent per year. The Bureau of Labor Statistics (BLS) calculated average growth of multifactor productivity of 0.8 percent per year over this same period. Calculating multifactor productivity is not as simple as the calculation we provided above. Some corrections the BLS includes in their calculation are (Bureau of Labor Statistics, BLS Handbook of Methods, Chapter 10, http://www.bls.gov/opub/hom/homtoc.htm):
BLS excludes the following outputs from real GDP growth: general government, nonprofit institutions, paid employees of private households, and the rental value of owner-occupied dwellings.
Real stocks are constructed as vintage aggregates of historical investments (in real terms) in accordance with an "efficiency" or service flow concept (as distinct from a price or value concept). The efficiency of each asset is assumed to deteriorate only gradually during the early years of an asset's service life and then more quickly later in its life.
The hours of employees of government enterprises are excluded. Also, the hours at work for each of 1,008 types of workers classified by their educational attainment, work experience and gender are aggregated using an annually chained (Tornqvist) index. The growth rate of total labor is therefore a weighted average of the growth rates of each type of worker where the weight assigned to a type of worker is its share of total labor compensation. The resulting aggregate measure of labor input accounts for both the increase in raw hours at work and changes in the skill composition (as measured by education and work experience) of the work force.
This last correction, weighting labor hours by skill, is probably the most serious. Earlier we said that multifactor productivity includes improvements in labor such as from better education or training. However, productivity increases arising from improving work skills through training or education may not show up in the multifactor productivity statistic reported by BLS. Consequently the BLS estimate of multifactor productivity growth should be smaller than our quick calculation.
![]()
Growth accounting is useful for identifying what contributed to the growth of output. But growth accounting does not explain why capital and technology grow at the rates they do. Growth models attempt to explain why expansion of the capital stock and economic growth are related.
The first model we study is the neoclassical growth model pioneered by Robert Solow in the 1950s (Robert M. Solow, "A Contribution to the Theory of Economic Growth," Quarterly Journal of Economics, February 1956, 65-94). Solow won the Nobel Prize in economics in 1987 for his work on economic growth.
Production conditions (i.e., supply rather than demand) generally dominate growth models. Growth models focus on the long-run trend in output, more commonly called potential or full-employment output, rather than the short-run booms and busts in which an economy cycles around its long-term trend. This allows us to avoid questions of business cycles, government stabilization policy, and unemployment and focus on the longer run issues of saving and investment policy. In fact, government policies designed to stimulate demand during a recession may have negative consequences for long-run growth. For example, tax cuts may "crowd out" investment spending as we will explain in later chapters.
The foundation of the neoclassical growth model rests on two assumptions:
1. Exogenously determined (i.e., determined by some process outside the model) labor supply, which grows at some given rate, n. Growth models do not assume households have more or fewer children as a country becomes poorer or wealthier (although the rate of population increase is generally lower in wealthier nations).
2. Some form of production function is assumed. In the neoclassical growth model the production function is assumed to exhibit constant returns to scale. Constant returns to scale simply means that if the labor force grows at 2% per year and capital grows at 2% per year then output also grows at 2% per year.
We present the neoclassical growth model in two parts. First, in Section A we apply the two assumptions in an analysis of a steady state. The surprising implication of this model is that the long-run steady state aggregate economic growth rate depends only on the labor supply (population) growth rate and not on the level of capital available to labor. Then in Section B we add equations for savings and investment to evaluate why poor countries do not catch up to the rich countries and if there is an optimal rate of savings and investment (called the Solow "Golden Rule").
Neoclassical growth models identify a steady state where the rates of change in output, capital and labor are constant. A steady state is something like an equilibrium condition. If there is no shock to the economy then markets will maintain a constant rate of growth. The question we ask is what determines the rate of growth.
| Steady State - a condition of constant rates of growth in economic measures. With no technological change, a steady state is represented by identical constant growth rates in the labor force, total output, and the level of capital. |
First we start with the growth accounting equation and assume that technology is constant, i.e., there is no productivity growth. In other words, in equation (2) ΔA/A = 0. Thus, we can simplify equation (2) as follows:
| ΔY = εK ΔK + εL ΔL Y K L |
(3) |
A steady state with no technological change implies that output per worker and the capital-labor ratio are constant. Capital must grow at the same rate as the labor force. If capital grows faster or slower than the quantity of labor then the economy is not in a steady state rate of growth. Another way of looking at it is if there is no technological change there should be no reason to increase or decrease the amount of capital every worker is given. New entrants to the labor force will be given a level of capital identical to all other workers.
If labor supply grows at some constant rate, n, then ΔL/L = n. Since the level of capital per worker is constant in this model of a steady state then capital must grow at the same rate as the labor force, or ΔK/K = n. Equation (3) can be rewritten:
| ΔY = εK n + εL n Y |
(4) |
Equation (3) doesn't tell us much because the elasticities of output with respect to capital, εK, and labor, εL, are unknown. We can give it some meaning by making a key assumption about the form of the production function, which determines the elasticities.
We assume the production function has constant returns to scale. Constant returns to scale simply means that if the labor force grows at 2% per year and capital grows at 2% per year (the capital-labor ratio in this steady state model is constant) then output also grows at 2% per year. Declining returns to sale in macroeconomic growth models implies that as the labor force grows (and the level of capital grows with it) a country would get poorer in terms of output (and real income) per worker. Total output would not increase as fast as the labor force. Thomas Malthus applied the concept of declining returns when he conjectured in 1798 (An Essay on the Principle of Population) that the world population would eventually outgrow the capability to produce food. Increasing returns to scale implies that output grows faster than the labor force. The only thing a country would need to do for its residents to become wealthier is to increase its labor force while maintaining the capital-labor ratio.
| Returns to Scale - the change in production that occurs when all resources are proportionately increased (increased by the same percentage). If labor, capital, and all other inputs to the production process increase by 1%, does output increase by more than 1% (increasing returns to scale), less than 1% (decreasing returns to scale), or exactly 1% (constant returns to scale)? |
When we assume constant returns to scale we can show mathematically (see Appendix C) that εK + εL = 1. Thus, equation (4) simplifies to reveal that the growth rate of output equals the growth rate of the labor force in steady state as shown in equation (5).
| ΔY = n Y |
(5) |
The surprising implication of this model is that the level of capital, K, has no affect on the long-term growth rate of an economy. The workers in one country can be loaded with the best tools and factories while another country's workers may be barely equipped, yet the steady-state aggregate economic growth rates of both countries can be identical if their populations are growing at the same rates. While output per worker will be greater for the country with the higher capital-labor (K/L) ratio, output per worker will not change in either country unless there is a change in total factor productivity. In other words, in steady state the economies of countries will grow at the same rate as their populations but the output of each individual worker remains unchanged. Economies can grow faster than their populations only if productivity improves.
In the opening of this section we made the simplifying assumption that technological change is assumed to be zero and total factor productivity remains unchanged. This is a very strong assumption. If instead technological change, A, in the production fucntion equation (1) and growth accounting equation (2) is some positive number then it should be obvious that economic growth is a combination of technological change and the growth rate of the labor force. This is more realistic but it doesn't change our primary observation that the level of capital does not affect the long-run growth rate of the economy in steady state.
The representation for technological change, A, in equation (1) is itself a strong assumption. This implies that technology advances at a constant rate over time and is neutral in that it relates to capital and labor in the same way. In other words, we get the same rate of productivity improvement regardless of the relative levels of capital and labor. The model can be modified to account for differences in technological change between the factors of production. For example, we could introduce a labor productivity factor, which would result in maintaining a steady state capital-output rather than capital-labor ratio (referred to as Harrod neutrality). We still get a similar result. Output grows at the same rate as the labor force plus the rate of technological change. The assumptions and result are perhaps more consistent with what we actually observe in most economies, but do not change the implications of the model.
So far we have established that the steady-state growth rate of aggregate output depends only on the rate of growth of the labor force (and technological change). The capital-labor ratio influences only the level and not the steady-state growth rate of an economy. For example, let's consider a rich and a poor country. Assume the per worker output of the rich country is 50% greater than that of the poor country. If the labor force growth rate of both countries is 2% per year then we can expect the aggregate output of both countries to double in 35 years. After 35 years the per worker output of both countries will remain unchanged and the rich country will still produce 50% more output per worker than the poor country.
| The 2% growth and doubling in 35 years is a simple rule-of-thumb that we get from the "Rule of 70." The rule of 70 states that the approximate number of years it takes a variable to double is 70 divided by the annual growth rate. |
Increasing the level of capital and the capital-labor ratio may not contribute to a permanently higher economic growth rate but it does give a short-term boost to output per worker and the standard of living. If countries can choose the amount of capital employed and raise or lower the capital-labor ratio is more capital always better? The next surprising result of our analysis is that a higher output per worker is not necessarily better. To see this result we need to expand the neoclassical model to include savings and investment in the Solow growth model.
A constant returns to scale production function was a key assumption that allowed us to simplify the growth accounting equation. In the Solow growth model we again put the constant returns assumption to use in a slightly different way.
A production function that represents constant returns to scale is represented in equation (6). We still assume that technological change, A, is zero. The constant returns production function, as noted earlier, implies that if we multiply both the quantity of labor and the quantity of capital by some positive number, z, then we also multiply output by z.
| z Y = f (z K, z L) | (6) |
If we assume the value of z is equal to 1/L, then we can convert the aggregate production function in equation (6) to a per worker production function in equations (7) and (8)
| Y = f (K/L, 1) L |
(7) |
| Y = f (K/L) L |
(8) |
So far nothing has changed from what was presented in the previous section. We have simply transformed the aggregate production function to a per worker basis, which is illustrated in Figure 3-3. Per worker output, Y/L, in Figure 3-3 is determined by the capital-labor ratio, K/L.
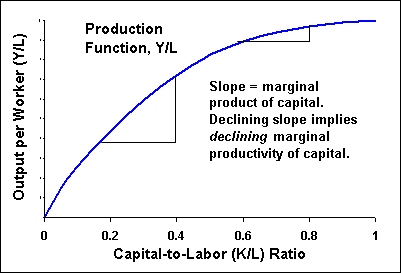
The production function has a positive slope because an increase in capital per worker, K/L, results in an increase in output per worker, Y/L. The slope of the production function represents the marginal product of capital. Each one unit increase in the capital-labor ratio increases output per worker by an amount equal to the marginal product of capital. The bowed shape of the production function implies diminishing marginal productivity of capital. Each incremental increase in capital with the amount of labor held constant (i.e., the capital-labor ratio increases) produces progressively smaller increases in output.
| Marginal Product - the change in the quantity of total output resulting from a unit change in a variable input, keeping all other inputs unchanged. The marginal product of capital is the change in output resulting from a 1 unit change in capital with the quantity of labor and other inputs held constant. |
Second, we assume the national savings rate is some fixed fraction of total output as shown in equation (9):
| S = s • Y | (9) |
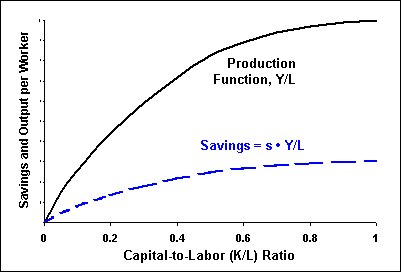
Savings on a per worker basis is shown in Equation (10). Output per capita is again plotted as a function of the capital-labor ratio in Figure 3-4. Output per capita is multiplied by the fixed savings rate, s, to obtain the lower dashed savings per capita line.
| S = s • Y L L |
(10) |
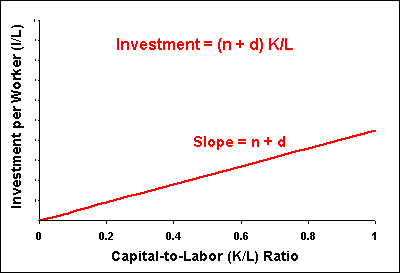
Third, investment is by definition equal to the change in the capital stock plus depreciation. We must not only equip new workers with capital but must also replace existing equipment that wears out or becomes obsolete. The change in the capital stock required to equip new workers is the labor force growth rate, n, times the current level of capital, or n • K. Let d represent the capital depreciation rate, or the fraction of capital that wears out each year. The amount of capital that must be replaced every year because of depreciation is the depreciation rate, d, times the level of capital, or d • K. Thus, total investment in steady state is represented by equation (11).
| I = n • K + d • K = (n + d) • K |
(11) |
Investment on a per worker basis is presented in equation (12) and illustrated in Figure 3-5.
| I = (n + d) • K L L |
(12) |
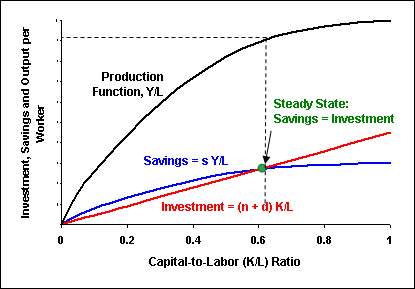
Fourth, we assume a steady state condition that savings equals investment. Steady state in Figure 3-6 is represented by the point the savings line crosses the investment line. If the capital-labor ratio is less than the steady-state level savings exceeds the level of investment needed to maintain this low capital-labor ratio. The extra savings is invested in new capital, which makes the capital-labor ratio increase until the steady-state point is reached. Similarly, if the capital-labor ratio is to the right of the steady state point we have the opposite situation. Savings is less than the amount needed to finance the investment required to replace depreciated capital and to equip new workers. The capital-labor ratio declines until the steady state level is reached.
One of the common empirical observations made when comparing economic growth rates across countries is the inverse relationship between wealth and population growth. The poorest countries generally appear to have the highest rate of population growth. The sociological reasons for high population growth rates among the poor are beyond the scope of this course. But we can use the Solow growth model to see why there is a relationship between population growth rates and wealth.
Consider two countries with identical production functions, savings and capital depreciation rates but different population growth rates. The identical production function assumption simply means that the same technology is available to both countries. Any differences in wealth are not due to political barriers that prevent the export of new technology from one country to another.
We can illustrate the effect of different population growth rates on wealth in Figure 3-7. Country B has a higher population and labor force growth rate than country A. This difference is reflected in the different investment lines in Figure 3-7 labelled IA for country A and IB for country B. The IB investment line is higher than the IA investment line because the slope, n + d, is higher, which reflects to the higher population growth rate, n. The consequence of the higher population growth rate is the output per worker is lower.
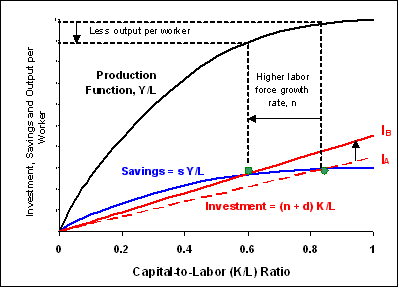
The logic is pretty simple. A given rate of savings will support a some amount of investment in capital goods. With more people continually entering the labor force in a country with the higher population growth rate the available capital goods must be spread more thinly. Each of the new and existing workers get fewer tools to work with and consequently produce less output. While the aggregate economic growth rate of a poor country may be greater than a wealthy country (because of the higher population and labor force growth rates), the poor country will remain poor relative to the wealthy country.
We can look at the effect of the savings rate on wealth in the same way we considered population growth except that we assume now the population growth rates of two countries are identical while the savings rates differ. A change in the savings rate, s, is required for an economy to move to a different capital-labor ratio. We show this in Figure 3-8 with a low savings rate country A and high savings rate country B. A poor country with low savings must operate at a low capital-labor ratio. A wealthy country with a higher savings rate may operate at a higher capital-labor ratio. A poor country can increase its wealth by increasing its savings rate thereby moving from a low K/L steady state to a higher K/L steady state over time.
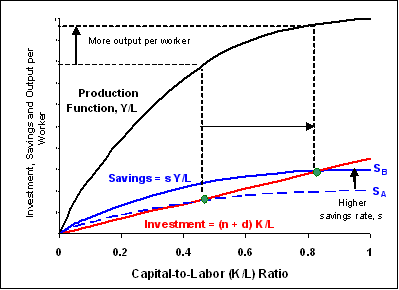
Why is one country poor and another wealthy? One answer lies in the capital-labor ratio. The wealthy country must have a higher capital-labor ratio than the poor country. But differences in capital-labor ratios and wealth do not affect aggregate economic growth rates in the neoclassical model. The steady state rate of growth of aggregate output of an economy is equal to the labor force growth rate. While one country may be poorer because of a high population and labor force growth rate, its aggregate economic growth rate may still be higher. But it won't catch up unless it can raise its capital-labor ratio.
For the poor country to catch up it must increase the level of capital employed per worker. However, the cruel dilemma of many poor countries is that increased investment requires savings to finance that investment. A higher savings rate means less current consumption. A higher savings rate may be impossible when only the basic necessities of living can be afforded in the poor country.
There is an incentive for capital to flow from the rich country to the poor country because of the declining marginal productivity of capital. If we were to move one unit of capital from the rich to poor country we would give up some small amount of output in the rich country but gain a much larger amount of output in the poor country. In the neoclassical model there is an economic incentive for convergence of the capital-labor ratios across countries.
We have seen an example of convergence with the impressive growth if the Asian "tigers" (such as Japan, Hong Kong, and Korea) over the last 50 years. The infrastructure of the east Asian nations had been decimated by World War II. Capital-labor ratios were comparatively very low. Through very high savings and investment rates their capital-labor ratios progressively grew. Economic growth came not just from labor force and technological change like other industrialized countries, but also because of an increase in their capital-labor ratios, which moved them up the production curve. This rapid capital intensification really can't go on forever. The declining marginal productivity of capital makes it uneconomical to sustain growth over the long term by increasing capital inputs alone. In fact the 1990s suggest that the envious growth rates of the Asian tigers has come to an end. The Asian tigers spent 40 years catching up and now they are just like us.
So why do some countries remain terribly poor? The answers are varied but often relate to barriers to the international flow of investment. For example:
While these explanations have appeal they still seem inadequate to explain differences in wealth across countries that last decades and even centuries. In the next section we will briefly describe a recent development in economic growth theory, the endogenous growth model, that attempts to explain why differences may persist and convergence may not occur.
If wealth increases with savings and investment is a higher savings rate always better? In our final application of the Solow growth model the surprising answer is no. It is not output per person that indicates wealth or welfare but consumption per person. We are supposedly better off when we can consume more.
There are only two things we can do with the output we produce: we either consume it or use it as investment to produce other goods and services. Consumption is the difference between total output and investment (or savings) as represented by equation (13).
| C = Y - I = Y - (n + d) • K |
(13) |
And consumption in equation (13) can be represented on a per worker basis by dividing through by the size of the labor force, L, in equation (14):
| C = Y - (n + d) • K L L L |
(14) |
Consumption per worker is shown in Figure 3-9 as the distance between output per worker and investment per worker.
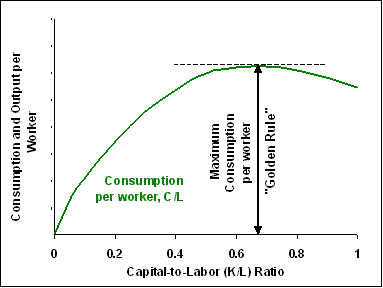
Notice in Figure 3-9 that as we increase the capital-labor ratio from a zero starting point the distance between output and investment gets progressively larger. Output rises faster than required investment and consumption per worker rises as the capital-labor ratio increases. At some point a maximum distance is reached and any further increases in the capital-labor ratio reduces the distance between output and investment per worker. Consumption begins to decline because of the curvature of the production function and the declining marginal productivity of capital. Maximum consumption occurs at the point where the slope of the per worker production function equals the slope of the investment line.
An increase in the capital-labor ratio has two opposing effects on consumption. First, a higher capital-labor ratio enables each worker to produce more output, which allows for an increase in consumption. Second, a higher capital-labor ratio requires more ongoing investment to replace worn out capital and equip new workers, which reduces consumption.
The implied level of consumption in Figure 3-9 is plotted in Figure 3-10, which reveals the effect of the trade-off between more output and more investment on consumption. Starting with low levels of capital, increases in the capital-labor ratio allow workers to consume progressively more. At some point a maximum is reached where the declining marginal productivity of capital is no longer large enough to support the level of investment required and consumption begins to decline. The point of maximum consumption is known as the Golden Rule capital-labor ratio (E.S. Phelps, "The Golden Rule of Accumulation: A Fable for Grown men," American Economic Review, 55, September 1965, 638-643). The Golden Rule suggests that it is possible to save and invest too much.

![]()
In the neoclassical growth model, the steady state growth rate of output per worker is exogenous, i.e. determined outside of the model. Increases in output per worker are only possible through technological change and increases in productivity. But the neoclassical model is silent as to the sources of technological change. Technological change is simply assumed to occur at some rate, which is independent of any macroeconomic variables (and is sometimes described as "manna from heaven").
Endogenous growth theory, first developed by Paul Romer and Robert Lucas (Paul Romer, "Increasing Returns and Long-Run Growth," Journal of Political Economy, October 1986, pp. 1002-1037, and Robert E. Lucas, Jr., "On the Mechanics of Economic Development," Journal of Monetary Economics, July 1988, pp. 3-42), focuses on the sources of technological change. Technological change is endogenous in their models, explained by the level of savings and investment. Countries that save more have greater increases in productivity and economic growth rates.
The key feature of endogenous growth models is that the marginal productivity of capital is no longer assumed to be decreasing. In the neoclassical model, the skills of the labor force do not increase as the level of capital increases and we have declining marginal productivity of capital. In endogenous growth models, the growing skills of the labor force may complement increases in capital.
The education, training, and skills of the labor force are referred to as human capital. As economies accumulate physical capital and become wealthier they devote more resources to education, training, and research and development. This investment in human capital increases productivity. If human capital increases at the same rate as physical capital then we could have constant or even increasing marginal productivity of physical capital.
We can identify the implications of the endogenous growth model by starting with a simple aggregate production function with the assumption of a constant rather than declining marginal productivity of capital. To simplify our model we also assume the number of workers does not change and accept the output and labor force growth rate relationship implied by the neoclassical model. This simplification means that the growth rate of aggregate output will be identical to the growth rate of output per worker in this model. We have in equation (15) a direct relationship between aggregate output and the level of physical capital.
| Y = α • K | (15) |
In equation (15) each additional single unit of physical capital, K, increases aggregate output, Y, by α units regardless of the level of capital employed. Because α does not depend on the level of capital we have constant marginal productivity of capital. The growth rate of output is equal to the growth rate of capital as shown in equation (16).
| ΔY / Y = ΔK / K | (16) |
We add to this model a representation for savings in equation (17). Aggregate savings is assumed to be constant at a fixed proportion, s, of total output.
| S = s • Y = s • α • K |
(17) |
Investment equals additions to the level of capital plus depreciation, equation (18), just as it did in the neoclassical model:
| I = ΔK + d • K | (18) |
In steady state aggregate savings must equal aggregate investment:
| s • α • K = ΔK + d • K | (19) |
Rearrange equation (19) to solve for the change in the physical capital stock:
| ΔK = s • α • K - d • K | (20) |
Divide both sides of equation (20) by K to get the growth rate of the physical capital stock:
| ΔK / K = s • α - d | (21) |
Substitute the solution for the growth rate of capital stock in equation (21) back into the output growth equation (15) and we get the result in equation (22) that the growth rate of output is a function of the savings rate:
| ΔY / Y = s • α - d | (22) |
The result that the growth rate of both total output and output per worker are a function of the savings rate is a significant departure from the neoclassical model. Savings affects long-run growth in the endogenous growth model because higher rates of saving lead to greater investment in human capital, which contributes to greater labor productivity.
Figure 3-11 presents a scatter plot of annual average savings rates (as a percentage of real GDP per worker) and real GDP per worker growth rates across countries over the period 1950 through 2000. The dashed linear regression line in Figure 3-11 indicates that each 10 percent increase in the average savings rate increases the growth rate of real GDP per worker by 0.45 percent. The United States falls right in the middle of the graph with an average savings rate of 19.2 percent and a real GDP per worker growth rate of 1.95 percent.
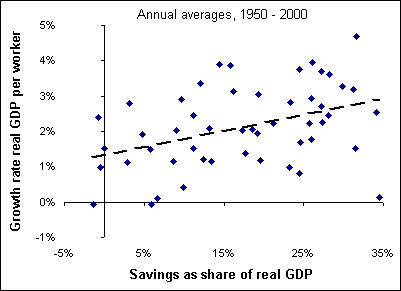
The neoclassical growth model suggested that standards of living of the poor and wealthy countries should converge on each other. The assumed declining marginal productivity of capital suggests that investment should migrate from the wealthy countries to the poorer countries, which have lower capital-labor ratios and higher marginal productivity from new capital investment. While there is some capital migration and some poor countries have converged on the wealthy in recent decades (in particular, the Asian tigers such as Hong Kong and japan), this usually seems the exception rather than the case.
The endogenous growth model provides an explanation for the absence of convergence. When there is a constant or even increasing marginal productivity of capital we should not expect the migration of investment from wealthy to poor countries as implied by the neoclassical model. Moreover. while there may be no barriers to the movement of physical capital from wealthy to poor countries, human capital cannot be transferred so easily. Differences in standards of living and economic growth rates may be sustained over time and we may not see convergence.
![]()
We saw that the neoclassical and endogenous growth models provided some conflicting implications with respect to convergence between poor and wealthy nations. The implications for the role of government policy in promoting long-run economic growth is also somewhat conflicting.
Government policies designed to promote long-run economic growth can generally be placed into one of three categories:
Policies that promote savings generally also stimulate capital investment and vice versa because of the impact on interest rates. A higher savings rate should lower real interest rates thus lowering the cost of borrowing for investment. Policies that stimulate investment would tend to raise the interest rate and provide an increased incentive for savings.
Most politicians and some economists have at some point chastised the American public for not saving enough. Perhaps the most significant forced-saving policy enacted in this country was Social Security. More recently, tax policies have been enacted to promote individual retirement accounts. Perhaps the biggest role in saving is played by the government. When a government spends more than it earns it reduces the rate of national saving. A household may be saving money but some of those funds go to the government for its own consumption spending.
Almost any policy designed to lower the cost of doing business will indirectly stimulate investment spending.
The neoclassical model suggests that policies designed to increase savings and investment spending may lead to a sustained increase in the standard of living, but only a temporary boost to economic growth. If a nation increases its rate of savings there are more funds available for investment. The capital-labor ratio rises with the resulting increase over time in the standard of living. But increasing savings and investment is not painless. Some current consumption must be foregone when the rate of saving increases. The payoff to a higher savings rate is not immediate but delayed. Moreover, the effect on the long-run economic growth rate is only temporary. With a higher savings rate the economy moves to a new steady-state capital-labor ratio and the economy returns to its previous long-run growth rate, although at a higher standard of living. If the savings or investment policy is not maintained then the opposite occurs. Investment falls off, we have a mini boom in consumption spending, the capital-labor ratio declines, and the economy returns to its original path with and standard of living.
The endogenous growth model, on the other hand, suggests policies that promote savings and investment may lead to a sustained increase in the growth rate of the economy, as long as those savings contribute to investment in new technology and human capital. Because growth in output and the standard of living is a function of the savings rate and human capital, the endogenous growth model provides a role for government policy that was absent in the neoclassical model. Governments can promote economic growth through policies that encourage savings as well as research and development, education, and health.
![]()
Production is a function of the economy's use of capital, K, labor, L, and a productivity index, A.
| Y = A • f(K, L, t ) | (A1) |
Take the total derivative of equation (A1) with respect to time, with df(.) used as a abbreviation for df(K,L,t):
| dY = dA f(K, L, t ) + A • df(.) dK + A • df(.) dL + A • df(.) dt dt dK dt dL dt dt |
(A2) |
We can make equation (A2) easier to read by abbreviating the time derivations:
| ΔY = ΔA f(K, L, t ) + dY ΔK + dY ΔL dK dL |
(A3) |
Divide equation (A3) through by Y, or the equivalent A • f(K, L, t):
| ΔY = ΔA + dY ΔK + dY ΔL Y A dK Y dL Y |
(A4) |
Multiply the second term on the right-hand side of equation (A4) by K/K and the third term by L/L.
| ΔY = ΔA + dY K ΔK + dY L ΔL Y A dK Y K dL Y L |
(A5) |
Finally, we can simplify equation (A5) by representing the term (dY•K)/(Y•dK) as the elasticity output with respect to capital and the term (dY•L)/(Y•dL) as the elasticity of output with respect to labor. For example the elasticity of output with respect to capital is the percent change in output, dY/Y, divided by the percent change in labor, dK/K.
| ΔY = ΔA + εK ΔK + εL ΔL Y A K L |
(A6) |
Equation (A6) is the basic growth accounting equation that decomposes growth in output into three parts:
Because elasticities are not observable (without knowing form of production function), they cannot be used in empirical analysis. But we can transform elasticities into income shares that can be calculated using government GDP/income survey data.
Assume a perfectly competitive market. The first-order condition of the microeconomic profit maximization problem is that inputs are paid the value of their marginal products, as represented in equation (B1).
| dY = r dK p |
(B1) |
The definition of the elasticity of output with respect to capital is presented in equation (B2):
| εK = dY K dK Y |
(B2) |
Substituting the profit maximization first-order condition that dY/dK = r/p from equation (B1) into equation (B2), we get the result in equation (B3) that the elasticity of output with respect to capital is equal to the income share to capital. The income share of capital equals the rents paid to the owners of capital for the use of their equipment, r K, divided by the value of total sales, p Y.
| εK = r K p Y |
(B3) |
The same procedure can be used for labor where the profit maximization first-order condition is dY/dL = w/p, where w is the wage rate.
If we assume constant returns to scale the elasticities of output with respect to capital and labor sum to 1. We start with the standard profit equation (C1).
| Profits = p • Y - r • K - w • L | (C1) |
Some basic microeconomics (that we will not show here) reveals that constant returns to scale implies total profits equal zero as in equation (C2).
| 0 = p • Y - r • K - w • L | (C2) |
| p • Y = r • K + w • L | (C3) |
Divide both sides of equation (C3) by p • Y:
| p • Y = r • K + w • L p • Y p • Y p • Y |
(C4) |
Equation (C4) simplifies to:
| 1 = r • K + w • L p • Y p • Y |
(C5) |
Equation (C5) shows that the income shares of capital (rK/pY) and labor (wL/pY) sum to 1. In the previous section we showed that elasticities of output are equivalent to income shares. Thus the elasticities of output with respect to capital and labor sum to 1.
| 1 = εK + εL | (C5) |
File last modified: June 1, 2005
© Tancred Lidderdale (Tancred@Lidderdale.com)